Material on this page is copyright © 2016 John W.F. Waldron
In getting from its original state A to some final deformed state B, a rock passes through an infinite series of very small steps of deformation, involving translation, rotation, dilation and distortion. We refer to this as the strain history. There are infinitely many routes that could be followed that could result in the same finite strain.
In analysing deformation, it is sometimes helpful to look at a very small part of the strain history, which is referred to as the incremental strain. Incremental strains may be represented by symbols such as δe or δγ
In strain theory, we sometimes go one step further and consider an infinitesimally small increment of strain, which is referred to as instantaneous strain or infinitesimal strain, effectively the derivative of the finite strain.
For example, if finite strain is represented by an extension e or a shear strain γ then an instantaneous strain is de or dγ.
The finite strain is the integral of the the infinitesimal strain, from the time of the formation of the rock to the present day.
de/dt and dγ/dt (also written , ) are strain rates - measures of the speed at which rocks flow.
What are the units of strain and strain rates? Strains and strain rates are dimensionless quantities, because they are ratios of two lengths. Therefore the units of strain rate are just "per second" or s-1. Sometimes structural geologists will say "strains per second". Typical geological strain rates are of the order of 10-14 strains per second (which works out to about 0.3 strains per million years).
Defining the complete strain history is a much larger challenge than measuring the finite strain. To keep thing simple at first we will continue to deal with only two dimensions - nothing flows in or out of our cross-section - 'plane strain'.
To describe progressive strain and flow, it's sometimes helpful to draw a field of small arrows representing the velocity field. We can also join these up to make longer arrows representing particle paths.
Particle paths are usually curved. Often there are one or two special particle paths that are straight. These have a special role in the generation of fabric in metamorphic rocks. They are known as flow asymptotes or flow apophyses. In linear algebra terms, they coincide with the eigenvectors of the deformation matrix.
At each moment in a strain history, the ongoing deformation is defined by instantaneous strain axes (or ISA: these are the instantaneous stretching and instantaneous shortening axes). Between them, there are usually lines of no instantaneous extension (LNIE).
All these lines may change orientation during the strain history. Except for special types of strain, the instantaneous directions of these lines do not coincide with their finite strain equivalents.
During deformation, material lines may move from the field of shortening to the field of extension or vice versa.
If rocks, and strain, were perfectly homogeneous, we would never be able to figure out strain histories because we would only see the end result, the finite strain. Fortunately, strain partitioning is common. In strain partitioning, different components of a rock record different parts of the strain history.
In coaxial strain the strain axes remain along the same material lines throughout the strain history: ie the strain axes do not rotate relative to the rock. As a result, the finite strain axes coincide with the instantaneous strain axes, and with the flow apophyses.
Note that although the strain axes don't rotate, other lines tend to rotate towards the stretching direction and away from the shortening direction.
Every increment of strain is non-rotational or pure strain. The vorticity of the deformation is zero.
The deformation matrix at every stage is a symmetric matrix. The flow apophyses, which are the same as the strain axes, coincide with the eigenvectors of the deformation matrix.
A diagram will show the effect of progressive pure shear on a rock with competent layers oriented initially perpendicular to s1
As strain starts, competent layers buckle to produce folds. Then, with increasing strain, limbs of the folds rotate from the field of incremental shortening into the field of incremental extension. Initial folds may become unfolded and undergo boudinage.
Geometries like these are extremely common in folded rocks - limbs that are boudinaged while the hinges are tightly buckled.
In noncoaxial flow, the strain axes and the rock rotate relative to each other. The instantaneous strain axes do not lie along the same material lines througout deformation.
In noncoaxial flow the deformation matrix is not symmetric and the flow apophyses (the eigenvectors of the deformation matrix) are not perpendicular.
If we think of non-coaxial flow as being broken down into an infinite number of tiny increments, we can mathematically consider it as consisting of separate increments of distortion (pure strain) and increments of rigid-body rotation
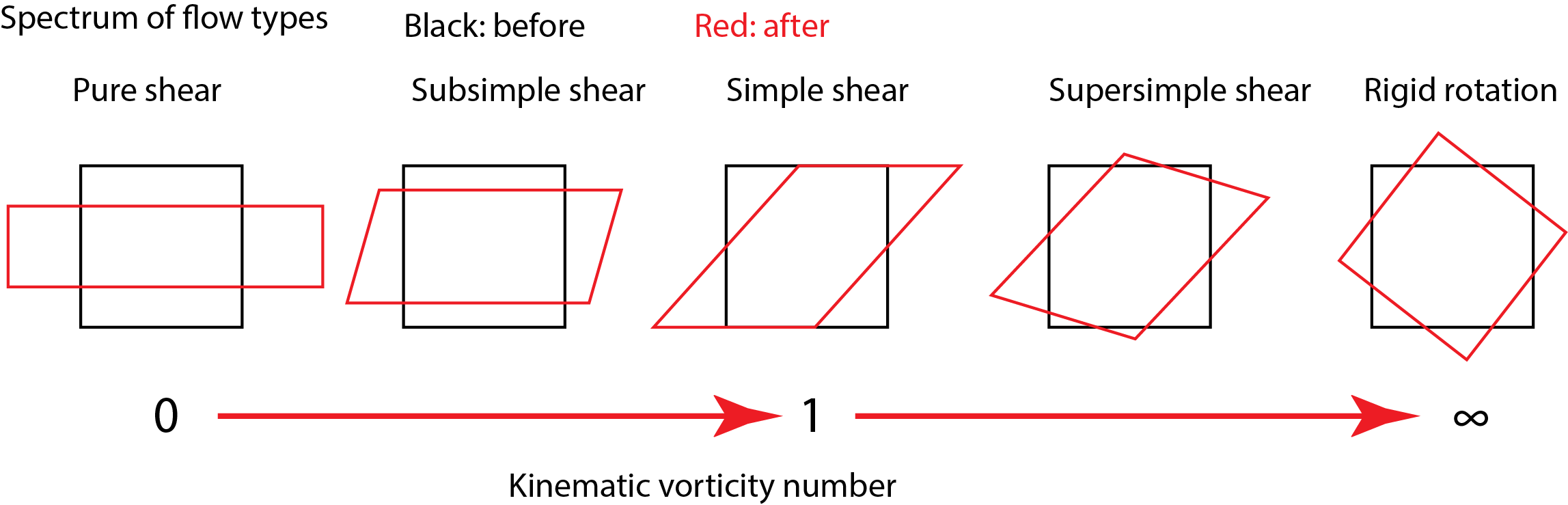
In dealing with non-coaxial deformations, it would be helpful to have a measure of the amount of rotation compared with distortion. To do this we define a quantity called vorticity. For a non-dilational deformation, the kinematic vorticity number is the ratio of the rotation rate (in radians per second) to the distortion rate (in strains per second).
It's possible to distinguish different categories of noncoaxial flow, depending on different amounts of vorticity.
Progressive pure shear is characterized by a kinematic vorticity number of zero.
Spectrum of flow types:

Simple shear is a special case of noncoaxial flow, but is important because an ideal shear zone develops by progressive simple shear.
In simple shear:
However, as finite strain increases:
Subsimple shear combines distortion with gradual rotation of the strain axes, and is intermediate between pure shear and simple shear. There are two flow asymptotes or apophyses, which coincide with the eigenvectors of the deformation matrix, but they are not at right angles and do not coincide with the strain axes.
The kinematic vorticity number equal to the cosine of the angle between the flow apophyses, and is therefore between zero and 1.
If the rate of rotation exceeds the rate of extension, everything rotates. No particle paths are straight and there are no flow apophyses. The deformation matrix has no real eigenvectors.
The vorticity number is greater than 1.
Supersimple shear describes the behaviour of relatively rigid particles in a shear zone, which tend to cause strain partitioning. The rigid particles capture the vorticity while the softer matrix captures the distortion.
Supersimple shear tends to produce features like delta porphyroclasts and helicitic (snowball) porphyroblasts.
Rotational deformation is much more complex in 3D than in 2D.
In 2D progressive deformation, rotation is about an axis perpendicular to the plane of section; any rotation axis is perpendicular to two strain axes.
In 3D, the rotational component of deformation can be perpendicular to two of the strain axes and therefore parallel to the third. When this is the case, the deformation is described as monoclinic. The 'before' and 'after' shapes of the strain ellipsoid have the same symmetry as a monoclinic crystal.
However, the rotation axis can be different from any of the strain axes. If this is the case, the strain symmetry is very low and the deformation is triclinic. Progressive triclinic strains (where rotation and distortion occur concurrently but on different axes) are extremely complicated to work with and require techniqes beyond the scope of this course.
The logarithmic Flynn plot, otherwise known as the Ramsay plot, comes into its own in dealing with strain histories. It turns out that is a constant incremental strain is applied, the finite strain follows a straight line path on the Ramsay plot.